area boxes for distributive property This process of writing these products as a sum uses the distributive property. Use the distributive property to re-write each expression as a sum. You may want to draw a rectangle It’s required by the NEC (National Electrical Code) to have junction boxes grounded, especially for metal ones. Grounding will always be a staple in every home’s electrical circuit because of the safety benefits. Note that non-metal junction boxes DO NOT need to be directly grounded.
0 · distributive property multiplying methods
1 · distributive property multiplication
2 · distributive property geometry
3 · distributive property examples
4 · distributive property area model
5 · distribution property using area name
6 · area names for distributional properties
Specialty Stainless offers top-notch stainless steel cabinetry, elevating your space with unparalleled quality and style. Our custom stainlees steel cabinets and doors are crafted with precision, utilizing high-grade stainless steel that enhances .
Distributive Property: Arrays and the Area Model . The Distributive Property. Definition: A number in a multiplication expression can be decomposed into two or more numbers. The distributive property can involve: multiplication over .This process of writing these products as a sum uses the distributive property. Use the distributive property to re-write each expression as a sum. You may want to draw a rectangle To use the distributive property, you need to break down the numbers into smaller parts. Once you have broken down the numbers, you can use the distributive property to multiply. To use the area model, draw a .value understanding using area models, partial products, and the properties of operations. Use models to make connections and develop the algorithm. STANDARD: NC.4.NBT.5
The distributive property of multiplication is used in all the methods we use to teach multi-digit multiplication. The area model, or box method, or window method for example is a .
Do your students struggle with multiplying large numbers? Help them learn multi-digit multiplication with strategies such as the area model, the box method, the partial products method, and the distributive property today! Click here to find .
Anytime you see a number outside of a set of parentheses within an expression, you can use the distributive property to help you to simplify by writing an equivalent expression .
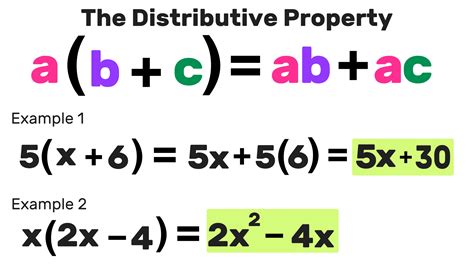
Once the lesson begins, I remind students of the area model and how to make arrays. I start with a simple array, such as 3 x 6. After students have created the array, then I ask them to brainstorm all the ways that we can break up the six. Simplify \(8 \left(x − \dfrac{1}{4}\right)\) using the distributive property and explain each step. Explain how you can multiply 4(.97) without paper or a calculator by thinking of .97 as 6 − 0.03 and then using the .
Instead of using the traditional way of expression called regrouping tens, it makes more sense to write it following the distributive property to a new learner, especially when larger numbers.Distributive Property: Arrays and the Area Model . The Distributive Property. Definition: A number in a multiplication expression can be decomposed into two or more numbers. The distributive property can involve: multiplication over addition (e.g., 6 x 47 = (6 x 40) + (6 x 7)) multiplication over subtraction (e.g. 4 x 98 = (4 x 100) – (4 x 2))
This process of writing these products as a sum uses the distributive property. Use the distributive property to re-write each expression as a sum. You may want to draw a rectangle
To use the distributive property, you need to break down the numbers into smaller parts. Once you have broken down the numbers, you can use the distributive property to multiply. To use the area model, draw a rectangle with the length and width representing the two factors.value understanding using area models, partial products, and the properties of operations. Use models to make connections and develop the algorithm. STANDARD: NC.4.NBT.5 The distributive property of multiplication is used in all the methods we use to teach multi-digit multiplication. The area model, or box method, or window method for example is a great way to show the distributive property.
Do your students struggle with multiplying large numbers? Help them learn multi-digit multiplication with strategies such as the area model, the box method, the partial products method, and the distributive property today! Click here to find out more. Anytime you see a number outside of a set of parentheses within an expression, you can use the distributive property to help you to simplify by writing an equivalent expression without parentheses. Let's look at an example.Once the lesson begins, I remind students of the area model and how to make arrays. I start with a simple array, such as 3 x 6. After students have created the array, then I ask them to brainstorm all the ways that we can break up the six.
Simplify \(8 \left(x − \dfrac{1}{4}\right)\) using the distributive property and explain each step. Explain how you can multiply 4(.97) without paper or a calculator by thinking of .97 as 6 − 0.03 and then using the distributive property. Instead of using the traditional way of expression called regrouping tens, it makes more sense to write it following the distributive property to a new learner, especially when larger numbers.Distributive Property: Arrays and the Area Model . The Distributive Property. Definition: A number in a multiplication expression can be decomposed into two or more numbers. The distributive property can involve: multiplication over addition (e.g., 6 x 47 = (6 x 40) + (6 x 7)) multiplication over subtraction (e.g. 4 x 98 = (4 x 100) – (4 x 2))This process of writing these products as a sum uses the distributive property. Use the distributive property to re-write each expression as a sum. You may want to draw a rectangle
To use the distributive property, you need to break down the numbers into smaller parts. Once you have broken down the numbers, you can use the distributive property to multiply. To use the area model, draw a rectangle with the length and width representing the two factors.value understanding using area models, partial products, and the properties of operations. Use models to make connections and develop the algorithm. STANDARD: NC.4.NBT.5 The distributive property of multiplication is used in all the methods we use to teach multi-digit multiplication. The area model, or box method, or window method for example is a great way to show the distributive property.Do your students struggle with multiplying large numbers? Help them learn multi-digit multiplication with strategies such as the area model, the box method, the partial products method, and the distributive property today! Click here to find out more.
Anytime you see a number outside of a set of parentheses within an expression, you can use the distributive property to help you to simplify by writing an equivalent expression without parentheses. Let's look at an example.Once the lesson begins, I remind students of the area model and how to make arrays. I start with a simple array, such as 3 x 6. After students have created the array, then I ask them to brainstorm all the ways that we can break up the six.
Simplify \(8 \left(x − \dfrac{1}{4}\right)\) using the distributive property and explain each step. Explain how you can multiply 4(.97) without paper or a calculator by thinking of .97 as 6 − 0.03 and then using the distributive property.
spc sheet metal
The sizing requirements for pull boxes, junction boxes, handhole enclosures, and conduit bodies exist to prevent conductor insulation damage. Those requirements are in 314.28, and they apply to all conductors 4 AWG and larger ( Fig. 1 ).
area boxes for distributive property|area names for distributional properties